Cancer Cell Mechanics

Mechanical interaction between the cell and its extracellular matrix (ECM) regulates cellular behaviors, including proliferation, differentiation, adhesion and migration. Cells require the three dimensional (3D) architectural support of the ECM to perform physiologically realistic functions. However, our current understanding of cell-ECM and cell-cell mechanical interactions is largely derived from 2D traction force microscopy, in which cells are cultured on a flat substrate. 3D traction force microscopy is emerging for mapping traction fields of single cells embedded in 3D gel, but current methods cannot account for the fibrous and nonlinear properties of biologically derived collagen gels. We are developing novel methods for 3D cell traction microscopy within collagen I gels and applying them to better understand disease processes such as cancer metastasis[1, 2].

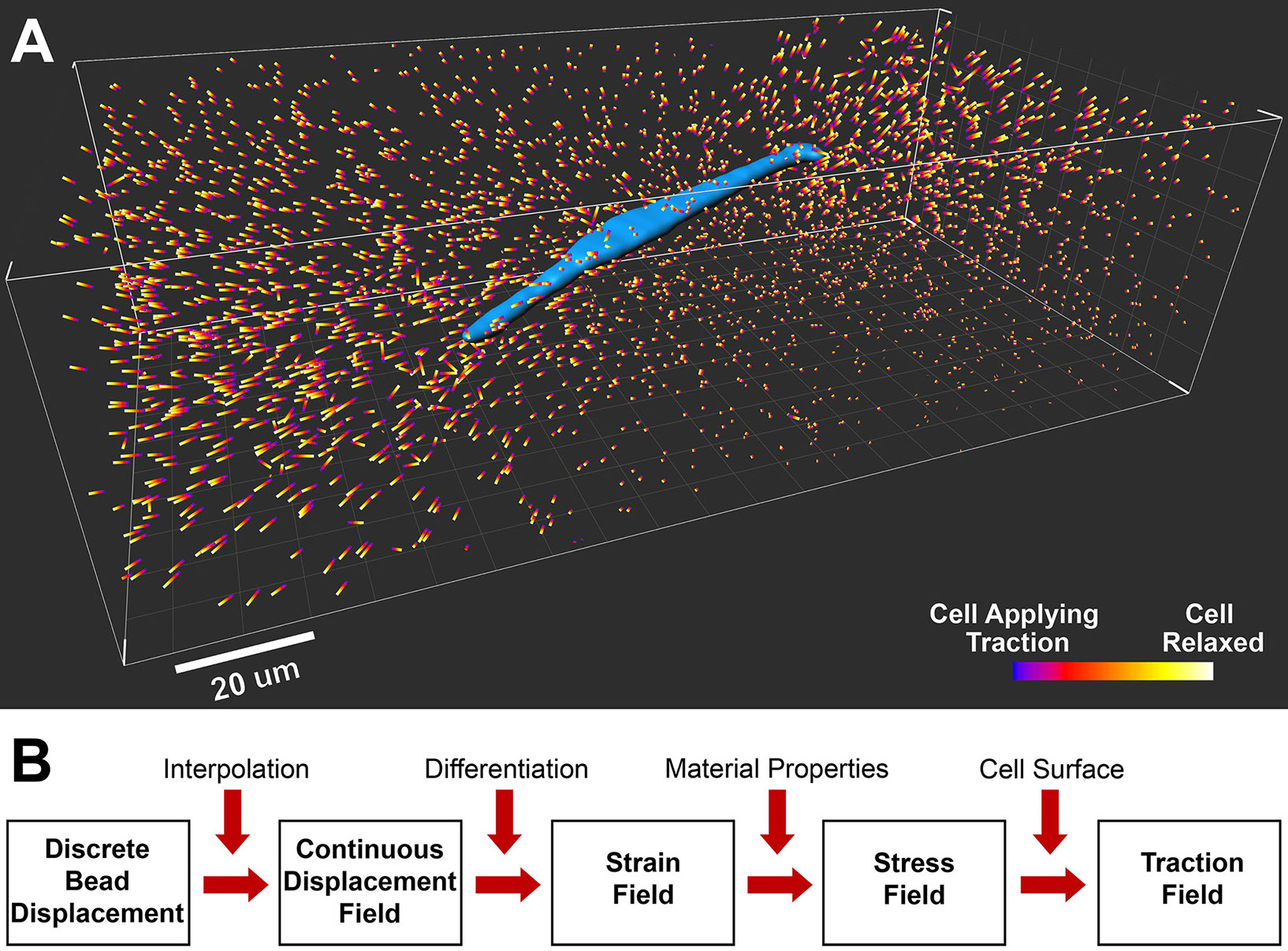
A breast tumor cell (blue, MDA-MB-231 cell line) is embedded in a type I collagen matrix. The colored bars are fluorescent bead displacements caused by relaxation of cell traction with a drug. B. Flow chart of a forward computation algorithm to translate the bead displacements into a 3D traction field on the cell surface.

The mechanical properties of the extracellular matrix have been shown to affect cellular behaviors including adhesion, differentiation, and migration. To better recapitulate the ECM in vivo¸ in vitro experiments increasing culture cells within biologically derived hydrogels such as collagen I with complicated nonlinear material behavior. Collagen I hydrogels are sensitive to polymerization conditions making an in situ method that can characterize gels as they are used for biological imaging experiments valuable. In addition, an in situ mechanical characterization method is ideal for understanding how some cells alter the material properties of their extracellular matrix. We are developing novel micro-indentation and imaging methods for characterization of biological hydrogels[2,3].

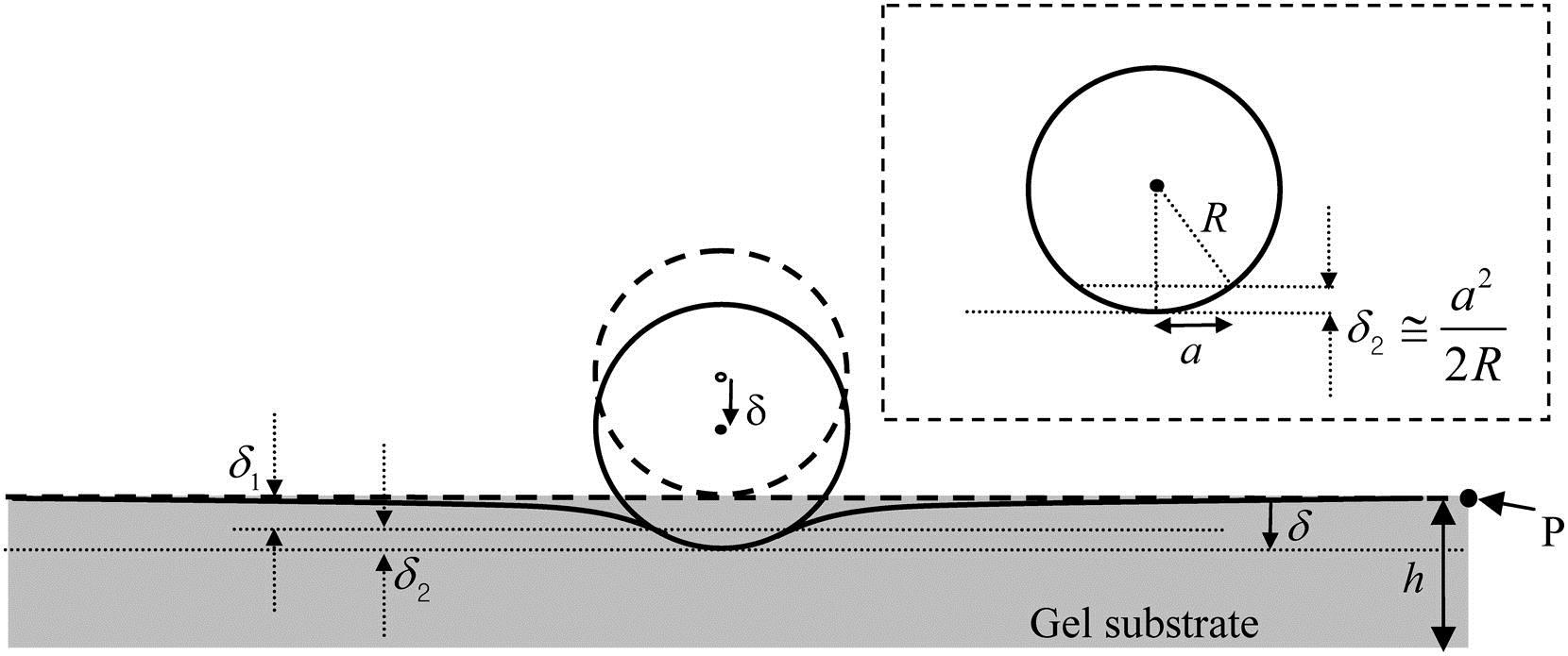
Diagram of a micro-indentation experiment for material characterization. The classical Hertz theory used to calculate Young's modulus from the indentation depth ? assumes that the gel thickness is an infinite half-space. Hertz theory therefore increasingly overestimates Young's modulus as gel thickness h decreases. A simple correction factor to the Hertz theory calculated modulus is provided in Long et al. [3] allowing accurate measurement of Young's modulus for gels with finite thickness.

Publications:

1. Beum Jun Kim, Pimkhuan Hannanta-anan, Michelle Chau, Yoon Soo Kim, Melody A. Swartz, and Mingming Wu, Cooperative roles of SDF-1a and EGF gradients on tumor cell migration revealed by a robust 3D microfluidic model, PLoS ONE 8(7): e68422. doi:10.1371/journal.pone.0068422 (2013).
2. Beum Jun Kim and Mingming Wu, Microfluidics for mammalian cell chemotaxis, invited review, Annals of Biomedical Engineering, 40, 1316-1327 (2012).